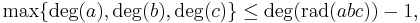Mason–Stothers theorem

The Mason–Stothers theorem, or simply Mason's theorem, is a mathematical theorem about polynomials, analogous to the abc conjecture for integers. It is named after W. Wilson Stothers, who published it in 1981,^[1] and R. C. Mason, who rediscovered it shortly thereafter.^[2]

The theorem states:

Let $a (t)$ , $b (t)$ , and $c (t)$ be relatively prime polynomials such that $a + b = c$ , with coefficients that are either real numbers or complex numbers. Then

$\max\{\deg(a),\deg(b),\deg(c)\} \le \deg(\operatorname{rad}(abc))-1,$

where $rad(f)$ is the polynomial of minimum degree that has the same roots as $f$ , so $deg(rad(f))$ gives the number of distinct roots of $f$ .^[3]

References

^ Stothers, W. W. (1981), "Polynomial identities and hauptmoduln", Quarterly J. Math. Oxford, 2 32: 349–370 .
^ Mason, R. C. (1984), Diophantine Equations over Function Fields, London Mathematical Society Lecture Note Series, 96, Cambridge, England: Cambridge University Press .
^ Lang, Serge (2002). Algebra. New York, Berlin, Heidelberg: Springer-Verlag. p. 194. ISBN 0-387-95385-X.

External links

Weisstein, Eric W., "Mason's Theorem" from MathWorld.
Mason-Stothers Theorem and the ABC Conjecture, Vishal Lama. A cleaned-up version of the proof from Lang's book.